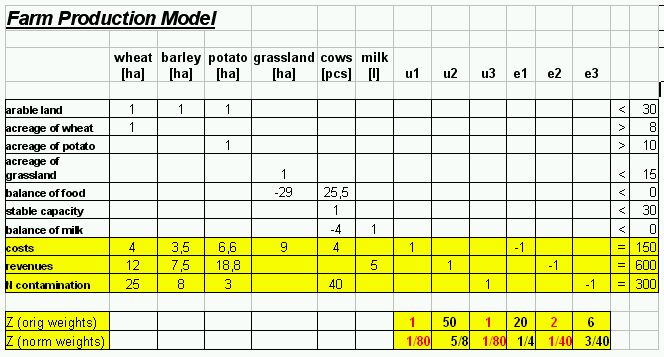
Let’s assume the farmers goals are: Costs $15000, revenues $60000 and N contamination 300 units. All these goals can be overachieved (with individually defined weights) or underachieved (with individually defined weights). On following Excel sheet both normalized an original weights are included, but the model is solved only with the normalized ones.

Solving these linear programming model using Linkosa software our manager obtains following optimal solution:
 

Optimal Solution of the Model FARM PRODUCTION Goal Programming Approach B
Min. value of the objective function Z (norm weights)
31,27
Structural variables				Constraints
Name	Value	Type		Name	Value	Slack
wheat [ha]	8	Basic		arable land	30	0
barley [ha]	0	Lower bound		acreage of wheat	8	0
potato [ha]	22	Basic		acreage of potato	10	-12
grassland [ha]	3,97	Basic		acreage of grassland	15	11,03
cows [pcs]	4,52	Basic		balance of food	0	0
milk [l]	18,08	Basic		stable capacity	30	25,48
u1	0	Lower bound		balance of milk	0	0
u2	0	Lower bound		costs	150	0
u3	0	Lower bound		revenues	600	0
e1	81,05	Basic		N contamination	300	0
e2	0	Lower bound
e3	146,8	Basic